Triality with Zometool

Introduction

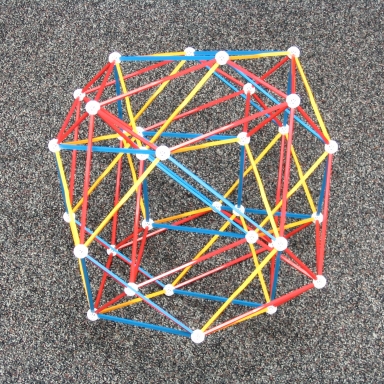
This page is about "triality" in the Zome System. Consider the followiong three Zometool models of the 16-cell:

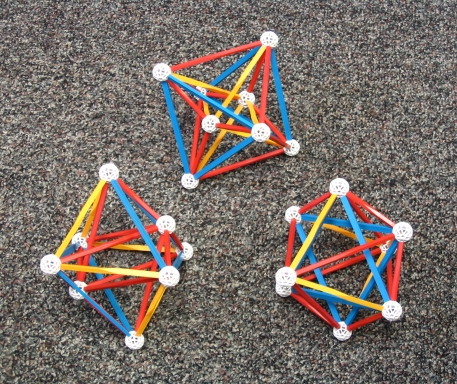
Zome Projections of the 16-Cell

Notice that each of these models is uses 8 connectors, 6 R1 struts, 6 R2 struts, 6 Y2 struts, and 6 B2 struts. Each model has two B2 equilateral triangles, each of which uses three connectors. For each model, the two remaining connectors lie on an axis of trihedral symmetry. For each model, there is a unique strut type among {R1,R2,Y2} which does not emanate from this trihedral axis. We therefore have a sort of triality, provided we ignore the distinction between yellow and red struts.

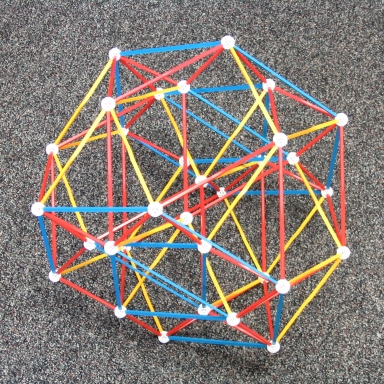
Zome Projection of the 24-Cell

Truncating the regular 16-cell to its edge midpoints yields the regular 24-cell. As a matter of fact, truncating any one of these models in this manner yields the same Zome model of the 24-cell. Thus, we have more evidence of a triality underlying these models. We shall see that this triality is a manifestation of the triality in the D(4) root system.

Quaternions and the 600-Cell

The purpose here is to see a somewhat non-intuitive construction of the 24-cell using the Zome System model of the regular 600-cell. This construction reveals that the binary icosahedral group is intimately related to D(4) triality.

The vertex sets for the three models of the 16-cell pictured above are imbedded in a particular way in the vertex set for the 600-cell, and there is some convenient notation for this set if one is willing to work with quaternions. Let Q={±1,±i,±j,±k} be the usual 8-element group of quaternions, where the elements satisfy Hamilton's equation,

i²=j²=k²=ijk=-1. Next, let T be the union of Q with the 16 quaternions obtained from ½(1+i+j+k) by applying all possible choices of signs of the coordinates. The set T is closed under quaternion multiplication and is known as the binary tetrahedral group. Moreover, the set T, as regarded as a subset of R⁴, comprise the vertices of a regular 24-cell.

Let b=½(1+√5) denote the Golden Ratio and a=½(1-√5) be its field-theoretic conjugate, and define a quaternion by

x:=½(-b1+i+aj). It is routine to verify that x⁵=-1 under quaternion multiplication. The elements of the binary icosahedral group consists of the union of the sets Q,xQ,x²Q,x³Q,x⁴Q.

Next, define the "binary icosahedral group" I to be the smallest group of quaternions containing i and ½(ai+j+bk), where b is the Golden Ratio and a is its field-theoretic conjugate. Then I contains T as a subgroup, and it also has the 96 quaternions which can be produced from

½(ai+j+bk) by applications of an arbitrary choice of signs and/or an even permutation of the four coordinates.

The elements of the groups Q, T, and I, as expressed here with quaternions, provide convenient notation for vertex sets of 4-dimensional regular polytopes. For example, the elements of Q coincide with the vertices of a regular 16-cell, the elements of T are the vertices of a regular 24-cell, and the elements of I are the vertices of a regular 600-cell. Moreover, since quaternion multiplication amounts to an orthogonal transformation, multiplying any of these sets by a fixed non-zero quaternion also yields vertex sets of the same polytopes.

If q is any non-zero quaternion, and G is any one of the groups {Q,T,I}, then let qG (respectively Gq) denote the set of all products of the form qx (respectively xq) where x is a quaternion in G.

The Zometool Models

In order to see the triality, consider the vertex set 2(1+i)I, obtained by multiplying each element of I by 2(1+i). This transformation is a composition with a "rotation" by π/4 with a dilation by √8. This set is invariant under the group generated by all permutations and all even numbers of sign changes in the four coordinates, but there are four orbits under this action. We may specify orbit representatives as

(-b²,a,a,a), (32 elements), (a-b,1,1,1), (32 elements), (a²,b,b,b), (32 elements), and (2,2,0,0), (24 elements). (For notational convenience, we denote the quaternion w+ix+jy+kz by (w,x,y,z). The last of these orbits is another image of the 24-cell; in fact, these 24 vectors coincide with the centers of the 24 octahedra of the 24-cell represented by T, thus exbihiting its self-duality.

One can see the triality by examining the first three orbits described above. Denote

[a]=(-b²,a,a,a)Q, [1]=(a-b,1,1,1)Q, and [b]=(a²,b,b,b)Q, the 16-cells left-induced by (-b²,a,a,a), (a-b,1,1,1), and (a²,b,b,b). Projecting down to 3-space using the mapping p, one obtains the three Zome models pictured above. This is where one should scrutinize the models very carefully. Only one of these three models has a pair of balls where only R1's and R2's meet. This model is the projection of [1]. The model with R2's and Y2's emanating from the trihedral axis is the projection of [b], and the remaining model is the projection of [a]. Evidently, p[b] has the vertex (b,b,b), p[1] has the vertex (1,1,1), and p[a] has the vertex (a,a,a).

Next, one can check that the union of the three vertex sets of [a], [1], and [b] coincide with the vertices of a 24-cell. Indeed, one has

[a]=½(a-b,1,1,1)(1,1,1,1)Q and [b]=½(a-b,1,1,1)(1,-1,-1,-1)Q. Since T is the union of Q with ½(1,1,1,1)Q and ½(1,-1,-1,-1)Q, the union of [a], [1], and [b] yields a 24-cell. In fact, one can check this with Zome, for truncating any one of these polychora to their edge midpoints yields the same projection of the 24-cell.

The Coxeter Graph D(4)